Lab 9: Operational Amplifiers and Feedback

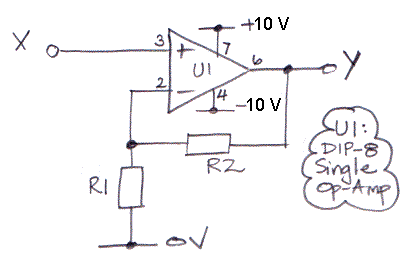
Part 1: Build the following non-inverting amplifier. Use R1 = 10 kΩ and R2 = 100 kΩ. Op-amp U1 can be the uA741 (original and classic), EL2044 (fast and rugged), or TL081 (low input bias current). Their data sheets are on the 29A web page. We give the DIP-8 pinout below.

The gain of an ideal op-amp is A = V_OUT/(V_IN+−V_IN−), where V_OUT is its output and V_IN+ and V_IN− are its non-inverting and inverting inputs respectively. What is the theoretical gain, G = Y/X, for this amplifier, assuming A is large? Apply a 1 Vpp sinusoid to X. Measure and plot the gain versus frequency from 10 Hz to 5 MHz. Give your plot a logarithmic frequency scale. Over what frequency range is the gain constant to within ±20%?

Part 2: Connect X to 0 V. Measure Y with a precision of ±1 mV. What is the input offset voltage of your op-amp? Connect X to 0 V with a 1 MΩ resistor. Measure Y once more. What is the input bias current of your op-amp?

Part 3: Apply a 1 Vpp sinusoid of frequency 400 Hz to X. Observe the amplitude on Y. Connect an 8-Ω speaker to Y. What is the amplitude of Y now? Is it sinusoidal? Connect a speaker transformer primary from Y to 0 V and connect your 8-Ω speaker to the secondary coil. What is the amplitude of Y now? What is the amplitude of the voltage across the speaker?

Part 4: Derive an equation for Y as a function of X for the following circuit. You may assume A is large.

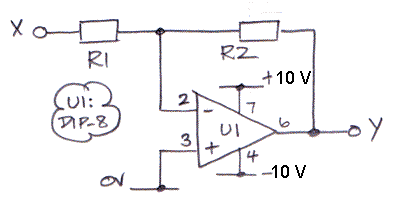
Build the above circuit with values of R1 and R2 that give a gain of −22. Apply a 1-kHz, 1-Vpp sinusoid to X and measure the amplitude at Y. Does your calculated gain match your measured gain?

Part 5: In the following circuit, R1 = R3 and R2 = R4. Derive an equation for Y as a function of X and W. You may assume A is large.

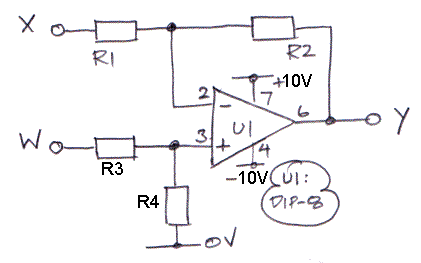
Arrange your equation in the form d(W−X) + c(W+X)/2. We call d the differential gain and c the common mode gain. In your solution, the common mode gain should be zero. Build the circuit with resistors of your own choosing. Devise a way to measure c and d with the help of your function generator. Do your calculations and measurements agree?