
Figure: The Basis of the Skin Effect.
The skin effect is the restriction of the flow of alternating current to the surface of a conductor. This restriction is caused by the alternating magnetic field that the current itself generates within the conductor. The higher the frequency of the alternation, the thinner the layer of conductor into which the current will be driven by this magnetic field. Let us begin by showing how Ampere's Law and Faraday's Law yield a differential equation for the current density with depth.

We see that the second derivative of current density with depth in the conductor must be proportional to the first derivative with time. We make two assumptions in coming to this conclusion. We assumed that the displacement current of Ampere's Law (with Maxwell's Addition) is negligible compared to the electrical current: ερ∂J/∂t << J. And we assumed the distance scale for changes in y is very much smaller than the distance scale for changes in z: δ << λ. We will continue with these assumptions, and later check that they hold true for the conductors and wavelengths we encounter in electronics. Let us proceed by deriving an expression for the skin depth, δ, which is the distance scale for changes in y.

Subject to our two assumptions, the amplitude of the current density will decrease exponentially with depth, in proportion to exp(−y/δ). The skin depth is δ = √(2ρ/ωμ). The following table gives some example values of skin depth for various conductors and frequencies.

We can now confirm that our assumptions about δ and ρ were correct. We assumed δ << λ. But λ = v / f, where v is the velocity of propagation of our wave along the conductor, and f = ω / 2π. We can solve for v using Maxwell's Equations and we will find that v ≈ 1/√(μ ε). Using Equation 7 above, and assuming copper is our conductor, we find that δ << λ when f <<< 2 × 1019 Hz. Our other assumption was that ερ∂J/∂t << J, the current density in the conductor. Looking at Equation 8 we see that differentiating by time multiplies by ω = 2πf. So our assumption is true in a copper conductor so long as f << 1 / (2περ) = 1.4 × 1016 Hz. Both our assumptions are valid so long as we work at frequencies below 1000 GHz.
The phase of the current density is not constant with depth. Indeed, at a depth y = πδ the phase of the current density is exactly opposite to the phase at the surface. The bulk current per unit width of conductor, which we called I, is the integral of the current density, J, with respect to depth. So now we consider the phase of I with respect J. We would like to know the phase of J at the surface with respect to I, and we would like to know the depth at which J is in phase with I.

Because the skin effect restricts I to a layer with thickness of order δ beneath the surface of the conductor, there is less conducting material to carry the current. In our infinite, planar conductor, the resistance of a unit width would be zero for direct current, because the cross-sectional area available is infinite. But for alternating current, the resistance is greater than zero.
In the following figure, we calculate the effective resistance per unit length of a one-meter width of our infinite, planar conductor. Our calculation uses argument of conservation of energy to deduce the skin resistance. Each infinitesmal layer of current beneath the surface of our conductor generates its own resistive heating. We add together the heat generated by these layers to obtain the total resistive heating per unit length of a unit width. Meanwhile, we have a bulk current flowing through the strip, and we define the skin resistance to be the ideal resistance that would produce the same total resistive heating if it carried the bulk current.
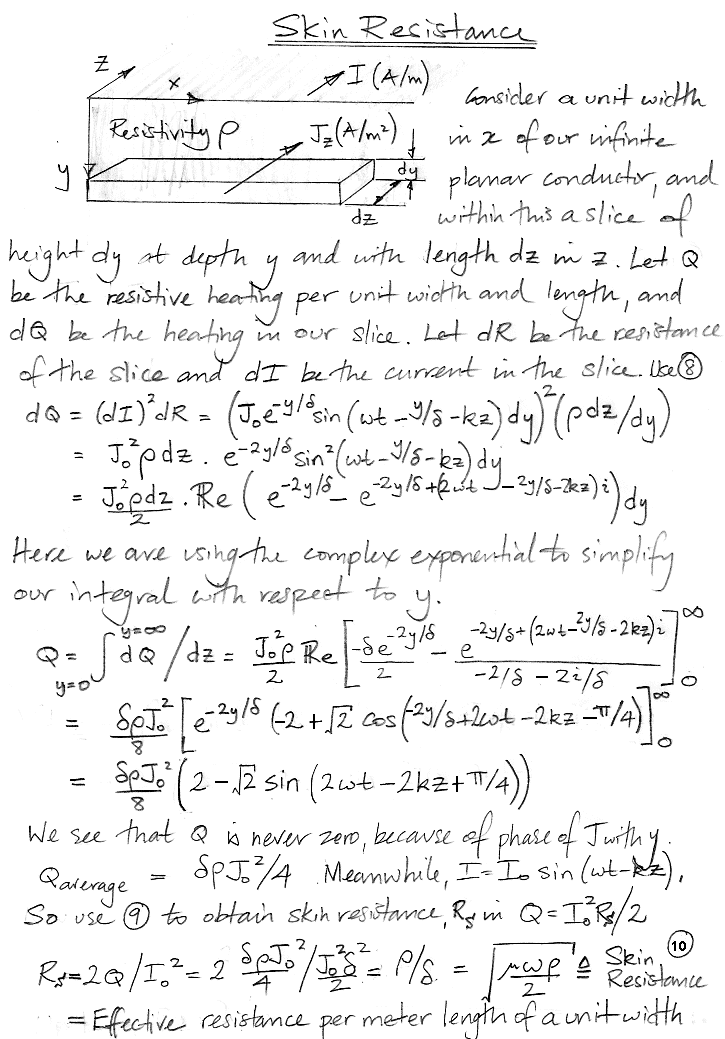
The skin resistance turns out to be the resistivity divided by the skin depth, which is the same value we would get if we assumed that the current flowed uniformly in a layer of depth equal to the skin depth. When we use our equation for skin depth, we find that the skin resistance is proportional to the square root of the resistivity, not the resistivity itself.
Our table of skin depths shows us that for frequencies greater than 1 MHz in copper, the skin depth is less than 64 μm. Suppose we have a solid copper wire with diameter much greater than the skin depth. We can calculate its effective resistance per unit length by dividing by the wire circumference.

In a 500-μm radius copper wire, Equation 11 holds for f >> 17 kHz. For copper, Equation 11 becomes R = 41 √f / r nΩ/m.